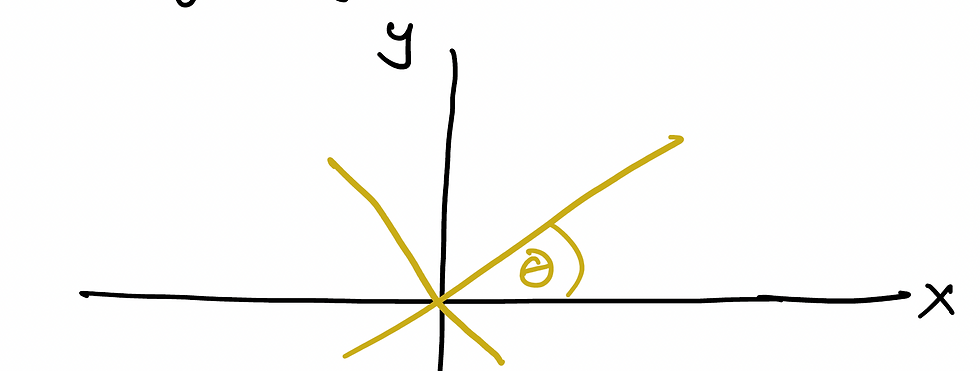
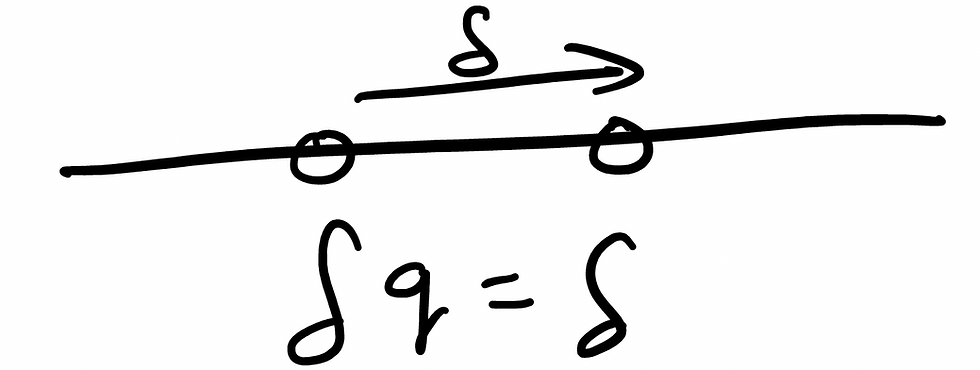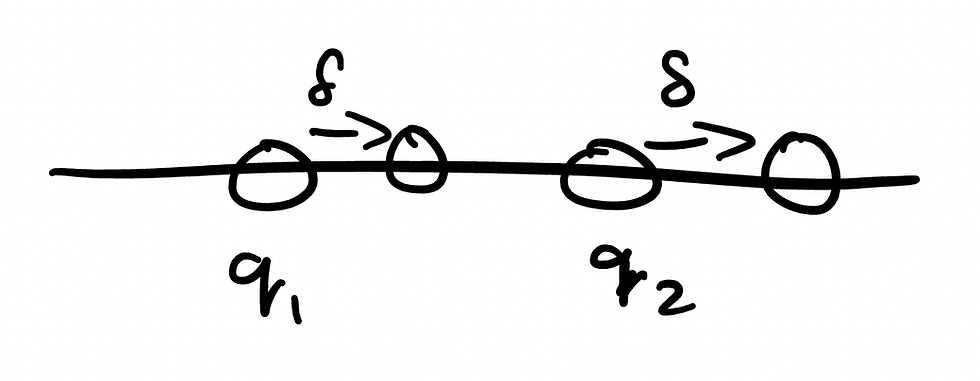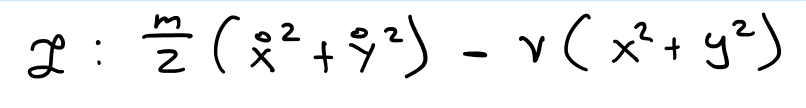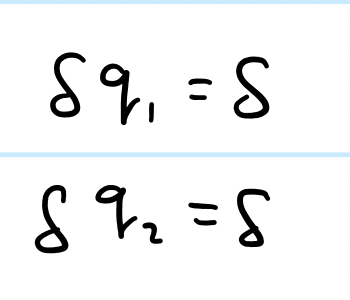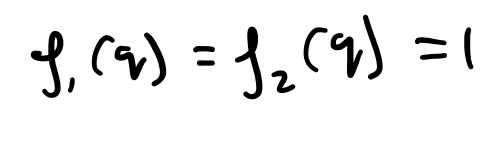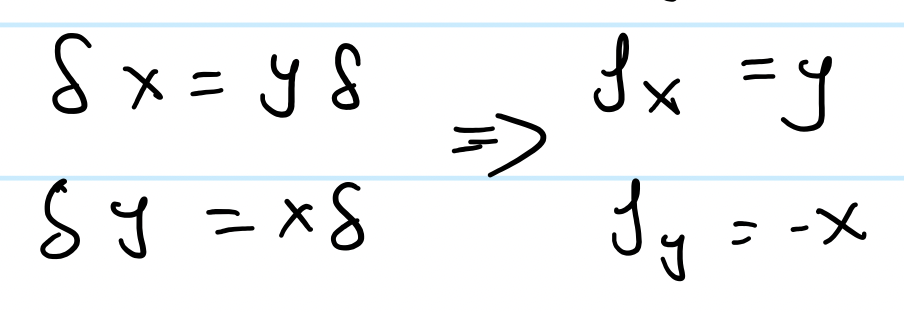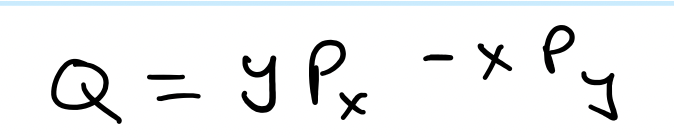- Adi Thakur
- Jun 30
- 1 min read
Hello, long time no see,
I am back after an extended, lazy sabbatical, and after a few years of putting this on the backburner, it's time to summon this page back to life.
I shall start with some basic notes on the basics of quantum physics, and try to make them even more basic than the basic notes themselves. Just like my coffee order, I will try and make it super basic (if that wasn't already clear).
I'm also keen on sharing some updates from arx.iv. That place is a godsend and I'd love to bring to light some of the newly released papers I find interesting on there.
Let's send this.